1.2 The Measures of a Wave: Velocity, Amplitude, Wavelength and Frequency
Like all other waves (waves in a string, water waves, sound, earthquake waves …), light and electromagnetic radiation in general can be described as a vibration (more general: a periodical change of a certain physical quantity) that propagates into space. The propagation is caused by the fact that the vibration at a certain location influences the region next to this location. For example in the case of sound, the alternating rarefaction and compression of air molecules at a certain location results in periodic changes in the local pressure, which in turn causes the movement of adjacent air molecules towards or away from this location.

Fig. 1: Formation and propagation of a wave in a string
The propagation is caused by the fact that the vibration at a certain location influences the region next to this location. For example in the case of sound, the alternating rarefaction and compression of air molecules at a certain location results in periodic changes in the local pressure, which in turn causes the movement of adjacent air molecules towards or away from this location.
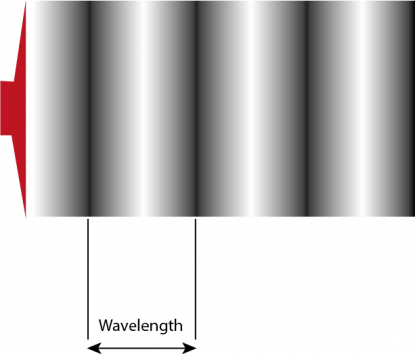 Fig. 2: Formation and propagation of a compression wave in air, a phenomenon colloquially called sound
Fig. 2: Formation and propagation of a compression wave in air, a phenomenon colloquially called sound
In the case of an electromagnetic wave, the mechanism of propagation involves mutual generation of periodically varying electric and magnetic fields and is far more difficult to understand than sound. Yet, the result can still be described as a periodic change of a physical quantity (the strength of the electric and the magnetic field) propagating into space. The velocity of this propagation is generally abbreviated with the letter c (unit: meters per second, m / s) and depends on the medium and nature of the wave (see Tab. 1 below).
| Sound | Optical (electromagnetic) radiation | |||
| at λ = 434 nm | at λ = 589 nm | at λ = 656 nm | ||
| in vacuum | – | 299792 km / s (n = 1) | 299792 km / s (n = 1) | 299792 km / s (n = 1) |
| in air | 340 m / s | 299708 km / s (n = 1.000280) | 299709 km / s (n = 1.000277) | 299710 km / s (n = 1.000275) |
| in water | 1500 m / s | 223725 km / s (n = 1.340) | 224900 km / s (n = 1.333) | 225238 km / s (n = 1.331) |
Tab. 1: Velocities of sound and light in air and in water. For optical radiation, the respective index of refraction is given in parenthesis
In order to describe the basic properties of a wave, the following quantities have been defined for all kinds of waves:
-
The amplitude is the maximum disturbance of the medium from its equilibrium. In the case of a wave in a horizontal string, this value is identical with half of the vertical distance between the wave’s crest and its trough.
-
The wavelength λ is the distance between two adjacent crests (or troughs) and is given in meters.
-
The period T of a wave is the time that elapses between the arrival of two consecutive crests (or troughs) at a certain location X. This definition is identical with the statement that the period is the time the vibration at X takes to complete a full cycle from crest to trough to crest. The period of a wave is given in seconds.
-
The frequency f of a wave is the number of vibration cycles per second at a certain location X. The unit of frequency is Hertz (Hz) and 1 Hz is the reciprocal of 1 second. As an example, a wave with a period T = 0.25 s takes ¼ of a second to complete a full vibration cycle (crest – trough – crest) at a certain location and thus performs four vibrations per second. Hence its frequency is f = 4 Hz. From this example, it is obvious that the period of a wave completely defines its frequency and vice versa. The relation between these quantities is given by f = 1 / T.
If we look at a wave as a process that is periodical in space and in time, we can regard the wavelength λ as the distance between two repetitions of the process in space and the period T as the ”distance” between two repetitions of the process in time.
A basic relation between wavelength, frequency and velocity results from the following consideration:
During the time span, a crest needs to travel the distance of one wavelength λ from location X to location Y. This time span is identical with the wave’s period T. And when a crest needs the time span T to travel the distance λ, its velocity c amounts to
| c = | λ | = λ f |
| T |
When a wave passes from one medium to another, its frequency remains the same. If the velocities of the wave in the two media differ, the wavelengths in the two media also differ as a consequence. Since the frequency of a wave does not depend on the medium the wave is passing, it is more convenient to use frequency instead of wavelength to characterize the wave. In acoustics, this is common practice – in most cases the pitch of sound is characterized by its frequency instead of its wavelength in a certain medium (for example air).
In optics, the situation is different: In most cases, wavelength is used instead of frequency although this leads to a certain complication: For example, green light has a wavelength of 520 nm in vacuum, but in water its velocity is smaller by a factor of 1.33 and thus, in water the same green light has a wavelength of only 520 / 1.33 = 391.0 nm. Hence, if we want to characterize a wave by its wavelength, we also have to state the medium for which the actual wavelength value is given. According to CIE regulations, which are applied throughout this tutorial, the term “wavelength” refers to “wavelength in air” unless otherwise stated. However, when applying the given wavelength figures to light passing through a medium other than vacuum, one should keep in mind that the light’s wavelength changes according to the following relation
| λMedium = | λVacuum | = | λAir × nAir |
| nMedium | nMedium |
with
| nAir = | cVacuum |
| cAir |
and
| nMedium = | cVacuum |
| cMedium |
nMedium is called the medium’s index of refraction and is more commonly used to specify the optical properties of a material than cMedium.